Singular cohomology
-
General Introduction
<]]> -
Search results
<]]> -
Online References
<]]> -
Paper References
<]]> -
Definition
Let
 be a topological space. Define the singular chain complex
be a topological space. Define the singular chain complex 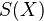 . Now
. Now 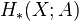 and
and  is the homology and the cohomology of
is the homology and the cohomology of 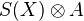 and
and 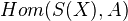 , respectively.
, respectively.This is a bifunctor, contravariant in
 and covariant in the coefficient group
and covariant in the coefficient group 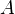 .<]]>
.<]]> -
Properties
<]]> -
Standard theorems
<]]> -
Open Problems
<]]> -
Connections to Number Theory
<]]> -
Computations and Examples
<]]> -
History and Applications
<]]> -
Some Research Articles
<]]> -
Other Information
Completely unverified, from MathOverflow: "Singular cohomology of $X$ can be expressed as sheaf cohomology if $X$ is locally contractible and $F$ is the sheaf of locally constant functions."
<]]> -
Comments Posted
<]]> -
Comments
There are no comments.